Gauss's Law
Homework Assignment Questions: 3, 4, *, 13, 15
Problems: 5, 9, 12, 23, 28, *, 58, **
from the FIFTH edition of Serway & Beichner
Q3: If there are more electric field lines leaving a gaussian surface than entering, what can you conclude about the net charge enclosed by that surface?
Q4: A uniform electric field exists in a region of space in which there are no charges. What can you conclude about the net electric flux through a gaussian surface placed in this region of space?
Q*. A point charge is placed at the center of an uncharged metallic spherical shell insulated from ground. As the point charge is moved off center, describe what happens to
(a) the total induced charge on the shell and
(b) the distribution of charge on the interior and exterior surfaces of the shell.
Q13. What would happen to the electrometer reading if the charged ball in Figure 24.20 touched the innter wall of the conductor? the outer wall?
Q15: Two solid spheres, both of radius R, carry identical total chares, Q. One sphere is a good conductor while the other is an insulator. If the charge on the insulating sphere is uniformly distributed throughout its interior volume, how do the electric fields outside these two spheres compare? Are the fields identical inside the two spheres?
24.5 Consider a closed triangular box resting withing a horizontal electric field of magnitude E = 7.8 x 104 N/C as shown here. Calculate the electric flux through
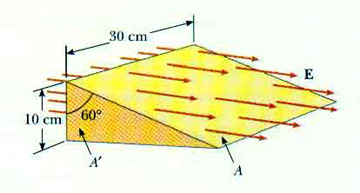
(a) the vertical surface,
(b) the slanted surface, and
(c) the entire surface of the box.
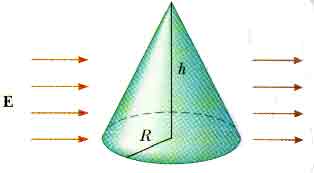
24.9 A cone of base radius R and height h is located on a horizontal table, and a horizontal uniform electric field E penetrates the cone, as shown here, in Figure P24.9. Determine the electric flux entering the cone.
24.12 Four closed surfaces, S1 through S4, together with the charges - 2 Q, Q, and - Q are sketched here, in Figure P24.12. Find the electric flux through each surface.
24.23 A charge of 170 microCoulombs, 170
C, s at the center of a cube of side 80 cm
(a) Find the total flux through each face of the cube.
(b) Find the flux through the whole surface of the cube.
(c) Would your answers to part (a) or (b) change if the charge were not at the center?
24.28 A solid sphere of radius 40.0 cm has a total positive charge of 26.0 microCoulombs, 26
24.* Consider a solid insulating sphere of radius b with nonuniform charge density
= C r for 0 < r, b. Find the charge contained within the radius when(a) r < b and (b) when r > b.
24.58 Two infinite, nonconduciting sheets of charge are parallel to each other as sketched here. The sheet on the left has a uniform surface charge density sigma
and the on on the right has a uniform charge density - sigma, -
24.** A slab of insulating material (infinite in two of its three dimensions) has a uniform positive charge density
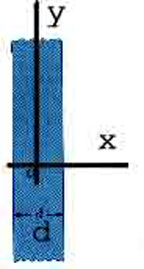
(a) Show that the electric field a distance x from its center and inside the slab is E = px/e.
(b) Suppose an electron of charge - e and mass m is placed inside the slab. If it is released from rest at a distance x from the center, show that the electron exhibits simple harmonic motion with a frequency
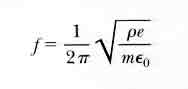
Return to Ch24 ToC
(c) Doug Davis, 2002; all rights reserved
