Statistics:High: 100%Average: 50%
Low: 0
Statistics:High: 100%Average: 50%
Low: 0
Possibly useful information:
s = si + vi t + (1/2) a t2 |
p = m v impulse = F impulse =
W = F • s = F s cos
A • B = A B cos A • B = Ax Bx + Ay By + Az Bz W = K = (1/2) m v2 E = K + U Wc = &endash; Fs = &endash; k x Us = (1/2) k x2 Ug = m g h = m g y |
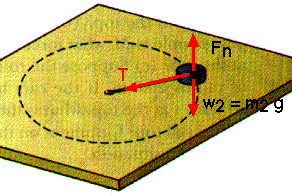
1. An air puck of mass 0.25 kg is tied to a string and allowed to revolve in a circle of radius 0.5 m on a frictionless, horizontal table. The other end of the string passes through a hole in the center of the table and a mass of 1.2 kg is tied to it. The suspended mass remains in equilibrium while the puck on the tabletop revolves. What are
(a) the tension in the string,
(b) the central force exerted on the puck, and
(c) the speed of the puck?
From the forces on the hanging mass, we can
immediately write T = (1.2 kg) (9.8 m/s2) T = 11.8 N This tension is also the central force exerted on the
puck; we call this the centripetal force. The puck also has the force of gravity, its weight
ww, pulling down on it and the normal
force Fn, pushing up on it. There is no
net force in the vertical direction; these two forces just
cancel. But, in the horizontal direction, the tension T
provides the net force on it; we call this force the
centripetal force. That centripetal force on the puck is connected
with the puck's mass and its speed: T = m v2 / r 11.8 N = (0.25 kg) v2 / 0.5 m v2 = (11.8 N) (0.5 m) / 0.25 kg v2 = 23.52 m2/s2 v = 4.85 m/s v = 4.85
m/s


2. An Atwood's machine has a 3.00 kg mass and a 2.00-kg mass at ends of the string . The 2.00-kg mass is released from rest on the floor, 4.00 m below the 3.00-kg mass.If the pulley is frictionless, what will be the speed of the masses when they pass each other?
KE
Wnet = W2 + W3 = - (2 kg) (9.8 m/s2) (2 m) + (3 kg) (9.8 m/s2) (2 m)
Wnet = 19.6 J
The small w's in the diagram -- w2 and w3 -- are the weights;
w2 = m2 g = (2.0 kg) (9.8 m/s2)
w3 = m3 g = (2.0 kg) (9.8 m/s2)
The capital W's in the equation above -- W2 and W3 -- are the amounts of work done on the 2.0-kg mass and the 3.0-kg mass by gravity.
Only the force of gravity does work on "the system". We need not -- can not -- consider the tension in the cord for that is an "internal force" just like the forces that hold the blocks together.
The blocks have a common velocity v,
KE = 0.5 (2.0 kg) v2 + 0.5 (3.0 kg) v2 = (2.5 kg) v2
KE = (2.5 kg) v2 = 19.6 J = Wnet
v2 = 7.84 m2 / s2
v = 2.8 m / s
We can describe this same situation now in terms of Conservation of Energy;
Ef = Ei KTot,f + UTot,f = KTot,i + UTot,i
K1f + K2f + U1f + U2f = K1i + K2i + U1i + U2i
(1/2) (2 kg) v2 + (1/2) (3 kg) v2 + (2 kg)(9.8 m/s2)(2 m) + (3 kg)(9.8 m/s2)(2 m) = = 0 + 0 + 0 + (3.0 kg) (9.8 m/s2) (4.0 m)
(1/2) (5 kg) v2 + 98 J = 117.6 J (1/2) (5 kg) v2 = 19.6 J
v2 = (19.6 / 2.5)J/kg
v2 = 7.84 m2 / s2
v = 2.8 m / s
3. A toy consists of a piece of plastic attached to a spring as shown here. The spring is compressed 0.025 m and the toy is released. If the mass of the toy is 0.120 kg and it rises to a maximum height of 0.75 m, estimate the force constant of the spring.Ei = Usi = (1/2) k x2 = (1/2) k (0.025 m)2 Later, at the top of its "jump", when its velocity is momentarily zero, there is only gravitational potential energy,
Ef = Ugf = m g hf = (0.120 kg) (9.8 m/s2) (0.75 m) = 0.882 J Since we expect energy to be conserved, this means
Ei = Ef (1/2) k (0.025 m)2 = 0.882 J
k = 2 822 N / m k = 2 800 N / m
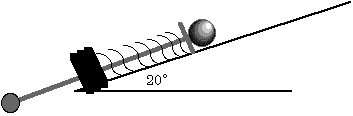
4. The launcher from a pin ball machine has a spring constant of k = 150 N/m. A pin ball, with mass of m = 0.10 kg, is launched by pulling back the spring and compressing it a distance of x = 0.05 m. The ball is then launched up a 20° incline as shown above. How far along this incline does the ball travel before itstops. Neglect friction. Neglect the mass of the spring and launcher. Neglect the rotational effects of the pinball.When the launcher spring is compressed 0.050 m, the 0.10 kg pinball is lower by 0.017 m so its gravitational potential energy is lower, too. We will take this as the reference position from which we measure the gravitational potential energy. Then,
Ugi = 0 Ki = 0
Usi = (1/2) k x2
Usi = (1/2) (150 N/m) (0.05 m)2
Usi = 0.1875 J
E = K + Ug + Us
Ei = Ki + Ugi + Usi
Ei = 0 + 0 + 0.1875 J
Ei = 0.1875 J
The spring is released, so Ugf = 0, and the pinball moves up the incline. Eventually it comes to rest, so Kf = 0. Where does this happen? It will be easier for us to calculate this in terms of the vertical distance ymax but it will be easier to describe or measure in terms of s, the distance along the incline. At this position,
Kf = 0 Usf = 0
Ugf = m g ymax
E = K + Ug + Us
Ef = Kf + Ugf + Usf
Ef = 0 + m g ymax + 0
Ef = Ei
m g ymax = 0.1875 J
(0.10 kg) (9.8 m/s2) ymax = 0.1875 J
ymax = 0.191 m
ymax = (s) sin 20o
s = ymax / sin 20o
s = 0.191 m / 0.342
s = 0.56 m
Remember, we have measured all our distances from where the pinball is when the launcher spring is compressed. It has moved 0.05 m from its initial position. So, as it moves up along the incline a distance of 0.56 m, it has moved 0.51 m from its initial position, before the launcher spring is compressed;
| Return to Course Calendar | Return to Dr D's Home Page | (C) 1998, Doug Davis; all rights reserved