Ch10

Chapter 10; Rotational Kinematics and Energy

| ToC, Chapter 10 | Course Calendar |

D10.1 It requires 6 seconds for a phonograph turntable initially rotating at 33 rpm to reach 45 rpm. Assume the angular acceleration is uniform. How many revolutions are made during this time?

D10.2 A ventilator fan is turning at 600 rpm when the power is cut off and it turns through 1000 revolutions while coasting to a stop. Find the angular acceleration and the time required to stop.

D10.3 A solid, uniform cylinder of 12 cm radius with mass of 5.0 kg is free to rotate about its symmetry axis. A cord is wound around the drum and a 1.2 kg mass is attached to the end of the cord. Find the acceleration of the hanging mass, the angular acceleration of the cylinder, and the tension in the cord.

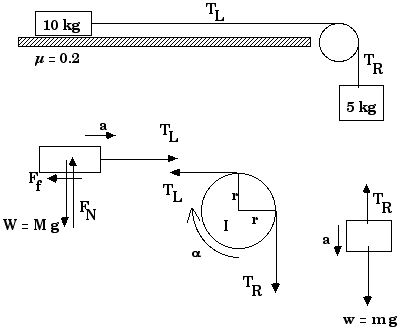
D10.4 A 10 kg block sits on a horizontal surface with coefficient of friction of 0.2 between the block and the surface. A string runs from this block over a wheel of radius 10 cm and moment of inertia of 2.0 kg m² and is attached to a hanging 5 kg mass. Find the acceleration of the masses, the angular acceleration of the wheel, and the tension in the string on each side.

D10.5 Assume a playground merry-go-round to be a uniform cylinder or disk of 150 kg and 1.8 m radius. What is its moment of inertia?

It is initially at rest when a 50 kg child, running at 4 m/s, in a direction tangential to the edge of the merry-go- round, jumps on. What is its angular velocity after the child sits down on the edge?

D10.6 What is the kinetic energy of tire with moment of inertia of 60 kg m² that rotates at 150 rpm?

D10.7 A disk rolls without slipping down a hill of height 10.0 m. If the disk starts from rest at the top of the hill, what is its speed at the bottom?

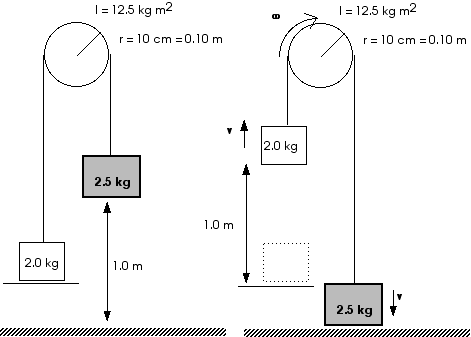
D10.8 An Atwood's machine is composed of a 2-kg mass and a 2.5-kg mass attahed to a string hanging over a wheel that has a radius of 10 cm and a moment of inertia of 12.5 kg-m². The 2.5-kg mass is initially 1.0 m above the floor. Use conservation of energy to find the speed of this mass just before it hits the floor.

D10.9 A basket of tomatoes of mass 20.0 kg is being hoisted by a windlass. The rope is wrapped around an axle that is a solid cylinder of wood having a radius of 0.1 m and a mass of 10 kg. The mass of the crank handles is neglibible. The operator lets go of the handle when the basket is 6 m above the ground. With what linear speed does the basket strike the ground?

D10.10 To demonstrate conservation of angular momentum a Physics professor stands on a frictionless turntable with a 2 kg mass in each outstretched hand. An assistant gives her a small initial angular velocity of 2 rad/s. She then drops her hands to her sides and her angular velocity increases dramatically. As a rough estimate, consider her arms to have mass of 5 kg each and to be 1 m long rods hinged at the axis of rotation. The rest of her body has an approximate moment of inertia of 0.55 kg m². Find her final angular velocity when the masses are 0.25 m from the axis of rotation. Calculate the initial and final values of the rotational kinetic energy and explain the cause of the difference in these values.

| Return to Calendar |